
Oh, that's because displacement is a vector. So if it's a vector, I put my vector symbol on it, and if this is a vector, somehow it has to become a vector. Even in one d, we have to think of it as a vector. One thing we've got to keep in mind is, even though we're doing one d, it is a vector. Instead of thinking it was an absolute final position in time, you can think of it as a continuous one if you wanted- some position past xi, some time past ti. It's really how far you go past the initial position over the time after ti. That's what delta x and delta t are, or if you want to think about it sort of in words, you could say it's the displacement past xi. If you wanted to write it out a little more gory, we could even say it's x final position minus x initial position over t final position minus t initial position. He's just sliding along, we can think about this time and this time and plot, or calculate, how far did it go, the displacement along the axis, delta x over the time. So if we go back to example two, and we have Hal here, and Hal is not rolling. We put average as a subscript when we mean the average velocity, and it's equal to the displacement, delta x, over that time interval, delta t. So what we're going to do- average velocity, we have our cursive v.
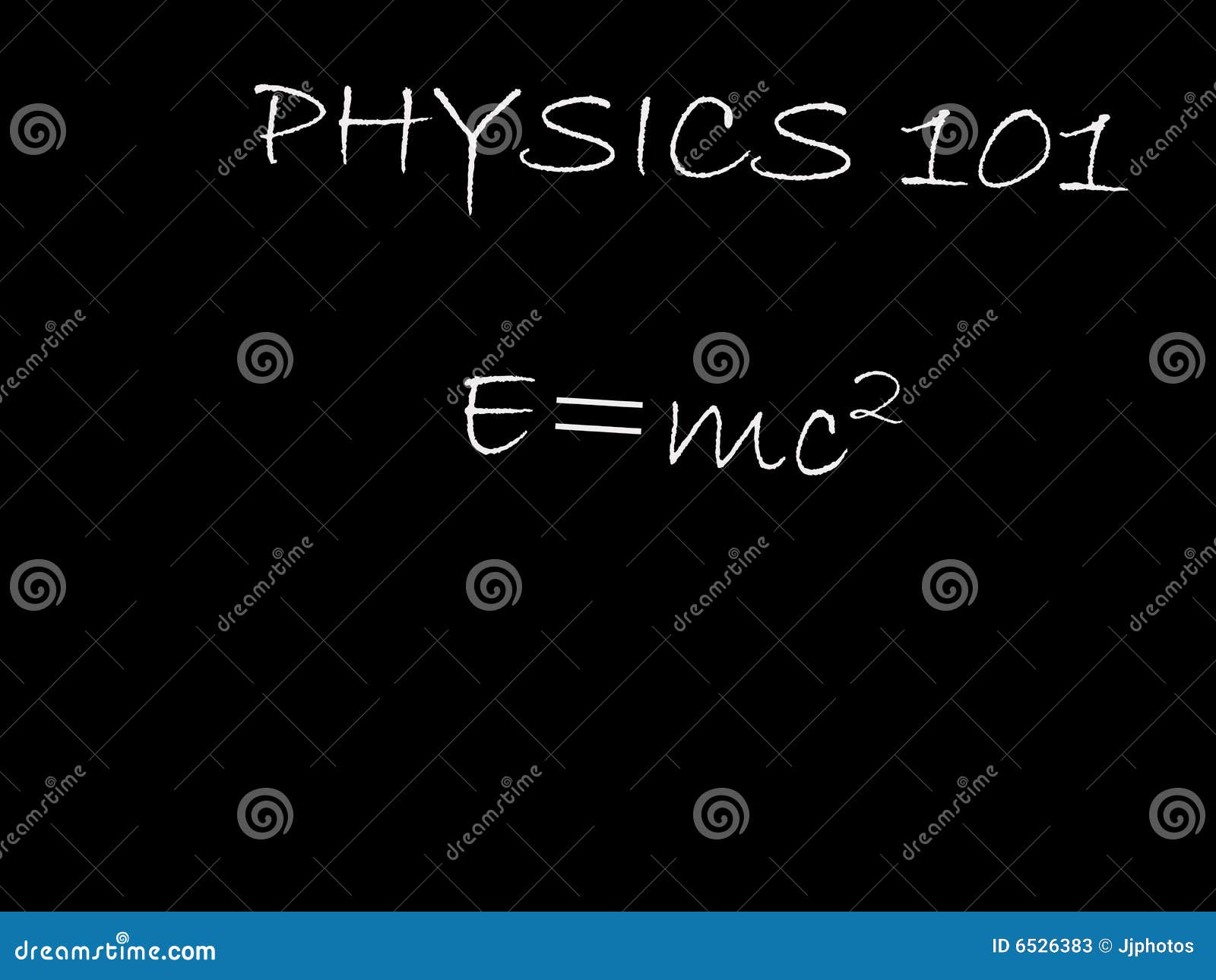

So let's just go for it here and define it. And just like displacements in position, delta t just means some final time, tf, minus some initial time, ti. You'll see why, but it depends on an interval of delta t. JASON HAFNER: Now we're going to talk about average velocity, and we're going to talk about it just during an interval delta t.


 0 kommentar(er)
0 kommentar(er)
